Mononobe-Okabe Theory
The coefficient Kae for active earth pressure is given by:
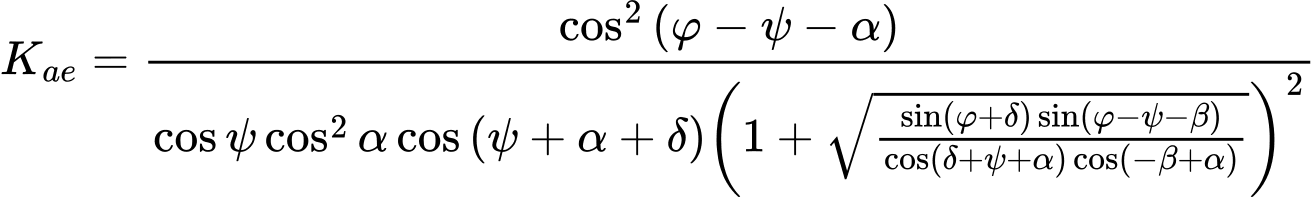
The coefficient Kpe for passive earth pressure is given by:
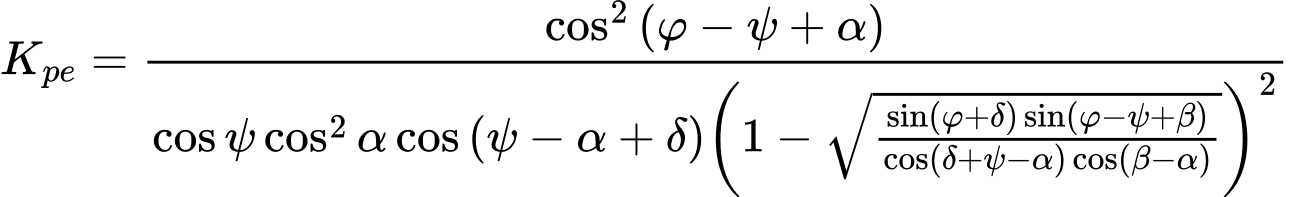
where: | γ | - | unit weight of soil |
H | - | height of the structure | |
φ | - | angle of internal friction of soil | |
δ | - | angle of friction between structure and soil | |
α | - | back face inclination of the structure | |
β | - | slope inclination | |
kv | - | seismic coefficient of vertical acceleration | |
kh | - | seismic coefficient of horizontal acceleration | |
ψ | - | seismic inertia angle |
Deviation of seismic forces ψ must be for active earth pressure always less or equal to the difference of the angle of internal friction and the ground surface inclination (i.e. φ - β). If ψ is greater, the program adjust the terrain slope to β = φ - ψ. In case of passive earth pressure the value of deviation of seismic forces ψ must be always less or equal to the sum of the angle of internal friction and the ground surface inclination (i.e. φ + β). The values of computed and modified angle ψ can be visualized in the output - in latter case the word MODIFIED is also displayed.
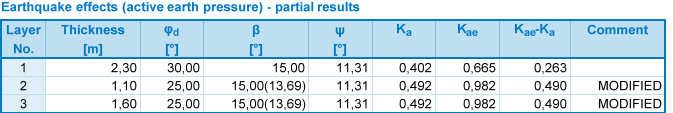Example of the program output
Literature:
Mononobe N, Matsuo H 1929, On the determination of earth pressure during earthquakes. In Proc. Of the World Engineering Conf., Vol. 9, str. 176.
Okabe S., 1926 General theory of earth pressure. Journal of the Japanese Society of Civil Engineers, Tokyo, Japan 12 (1).