Pile Horizontal Bearing Capacity - Brom’s Method
Analysis of a single pile according to Broms is described in Broms, 1964. This method exclusively assumes a pile in the homogeneous soil. Thus the analysis method does not allow for layered subsoil. The type of analysis of the pile horizontal bearing capacity is specified in the "Settings" frame, tab "Piles".
When adopting the Broms method for the analysis of horizontal bearing capacity the program disregards up now input soil layers. The soil parameters are specified in the "Horizontal bearing capacity" frame based on the type of soil (cohesive, cohesionless).
The input parameters for the analysis of pile horizontal bearing capacity are the pile material characteristics (modulus of elasticity and strength of a given material), pile geometry (pile length l and its diameter d), and also the pile load due to shear force and bending moment.
The coefficient of pile stiffness β for cohesive soils is given by:
![]()
where: | E*I | - | bending stiffness of pile section [MNm2] |
kh | - | modulus of subsoil reaction [MNm3] | |
d | - | diameter of a single pile [m] - in case of a pile with a circular variable cross-section the calculation of parameter β assumes a constant value of the pile diameter d1 input in the "Geometry" frame |
The coefficient of pile stiffness η for cohesionless soils follows from:
![]()
where: | E*I | - | bending stiffness of pile section [MNm2] |
nh | - | coefficient of soil modulus variation [MNm3] |
The program automatically determines whether to consider a long or a short pile based on the values β*l (for cohesive soils) and η*l (for cohesionless soils), respectively. Because literature offers different criteria for different types of piles, the program allows the user to define them. For an intermediate pile length the verification analysis considers both short and long piles and then the program automatically chooses the result with the lowest value of the pile horizontal bearing capacity Qu.
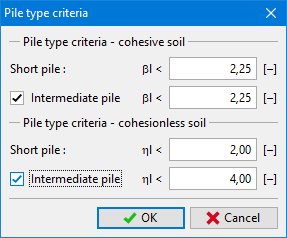 Dialog window "Pile type criteria"
Dialog window "Pile type criteria"
Type of pile criteria (long, short, medium) are considered according to the following conditions:
- free head: for long piles, it holds β*l > 2,5; for short piles then β*l < 2,5
- restrained: for long piles, it holds β*l > 1,5; for short piles then β*l < 1,5
Type of pile (pile head support) can be considered in two ways:
- free head - rotation at pile head is not constrained
- restrained - pile is constrained against rotation at its head. In such cases we typically deal with piles that are part of a planar pile grid or a pile group.
Another important input parameter is the flexure bearing capacity. This quantity is automatically back-calculated by the program using the following relation:
![]()
where: | Wy | - | section modulus of the pile section [m3] |
f | - | strength of the pile material [MPa] | |
γk | - | reduction coefficient of cross-section strength [-] - the cross-section bearing capacity is according to different standards pre-multiplied by different safety coefficients. This coefficient enables to adapt the program to these standards. |
In case of a steel-reinforced concrete pile the flexure bearing capacity, Mu depends on the amount of designed steel.
The reduction coefficient of bearing capacity γQu reduces the overall magnitude of the single pile horizontal bearing capacity as:
![]()
where: | Qu | - | horizontal bearing capacity of a single pile [kN] |
γQu | - | reduction coefficient of bearing capacity [-] |
The result of an analysis is horizontal bearing capacity of a single pile Qu, respectively Qu,red and displacement of a pile at the terrain surface u.
Literature:
[1] BROMS, BENGT. B.: Lateral Resistance of Piles in Cohesive Soils. Proceedings of the American Society of Civil Engineers, Journal of the Soil Mechanics and Foundations Division, Vol. 90, SM2, 1964.
[2] BROMS, BENGT. B.: Lateral Resistance of Piles in Cohesionless Soils. Proceedings of the American Society of Civil Engineers, Journal of the Soil Mechanics and Foundations Division, vol. 90 SM3, 1964.